Binding Positrons
Positron Binding
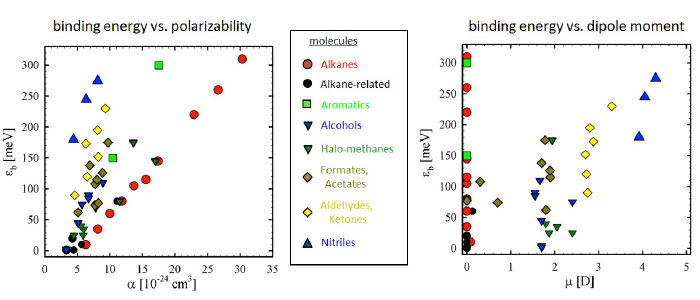
Positrons bind to most molecules. Binding energies have now been measured for over 100 molecular species including alkanes, cycloalkanes, halo-methanes, alcohols, aldehydes, ketones, formates, acetates, nitriles, and aromatics. Binding energies range from a few millielectron volts to ≥ 300 meV with typical uncertainties ≤ +/- 10 meV. Generally, the measured binding energy depends on the molecular polarizability, dipole moment and numbers of pi bonds, but molecular geometry (e.g., isomerization) can also be important.
While electrons bind weakly to many molecules, positron binding is a factor of 10 – 100 times stronger. Two contributing factors are that positron-electron interactions are attractive, and if there is a permanent dipole moment, the negative end of the dipole is nearer the periphery of the molecule where the positron can enjoy a lower potential energy. Both effects lead to stronger positron binding.
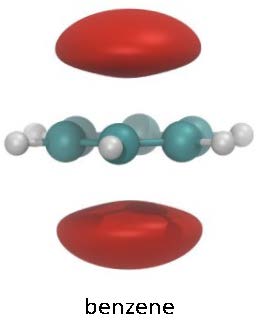
Increased molecular polarizability, permanent dipole moment and the number of molecular pi bonds all lead to stronger binding, and to localization of the bound-state positron wavefunction. In the case of polarizability, the positron surrounds the molecule rather uniformly, while a permanent dipole attracts the positron to that location, and pi bonds attract the positron to the region of the electron orbitals (typically away from the repulsive atomic cores). This pi-bond effect is illustrated in the figure at the upper right for benzene. Geometry of the atomic cores can also play a role; and so, for example, chlorine substitution in hydrocarbons leads to deeper binding if the chlorine atom is placed near the center of the molecule. The lower figure at the right compares the wave functions of n-propyl chloride and isopropyl chloride, where the positron wave function is localized due to the dipole moment. The binding energies are 97 and 113 meV, respectively.
Model calculations by Swann and Gribakin and by Tachikawa and colleagues capture many of these effects. A recent ab initio many-body theory of positron binding by Green and collaborators elucidates the important contribution to positron binding due to electron-positron correlations and virtual positronium formation. It yields good, quantitative agreement with experimentally measured binding energies for a wide range of molecules.
Further Reading:
Enhancement of positron binding energy in molecules containing p bonds, J. R. Danielson, S. Ghosh, and C. M. Surko, Phys. Rev. A206, 032811 (2022).
Influence of geometry on positron binding to molecules, J R Danielson, S Ghosh and CM Surko, J. Phys. B 54, 225201 (2021 ).
Positron-molecule interactions: Resonant attachment, annihilation, and bound states, G. Gribakin, J.Young, and C. Surko, Rev. Mod. Phys. 82, 2257 (2010).
Many-body theory of positron binding to polyatomic molecules, J. Hofierka, B. Cunningham, C. M. Rawlins, C. H. Patterson, D. G. Green, preprint (2021)
Effect of chlorination on positron binding to hydrocarbons: experiment and theory, A. R. Swann, et al., Phys. Rev. A 104, 012813 (2021)
Positron-electron correlation-polarization potential model for positron binding in polyatomic molecules, Y. Sugiura, et. al., J. Comp. Chem. 41, 1576 (2020).
Effect of molecular constitution and conformation on positron binding and annihilation in alkanes, A. R. Swann and G. F. Gribakin, J. Chem. Phys. 153, 184311 (2020).
Calculation of positron binding and annihilation in polyatomic molecules, A. R. Swann and G. F. Gribakin, J. Chem. Phys. 149, 244305 (2018).
Interplay between permanent dipole moment and polarizability in positron-molecule binding, J. R. Danielson, et al., Phys. Rev. A 85, (2012).
Interplay between permanent dipole moments and polarizability in positron-molecule binding, J. Danielson et al., Phys. Rev. A 85, 022709 (2012).
Dipole Enhancement of Positron Binding to Molecules, J. Danielson et al., Phys. Rev. Lett., 104, 233201 (2010).
Bound states of the positron with nitrile species with a configuration interaction multi-component molecular orbital approach, M. Tachikawa et al., Phys. Chem. Chem. Phys. 13, 2701 (2011).